1. Chúng minh hàm số đồng biến trên các khoảng \(\left( { – \infty ; – 1} \right)\) và \(\left( { – 1; + \infty } \right)\) .
2. Xét tính chẵn – lẻ của hàm số \(f(x) = \dfrac{{\sqrt {5 + 2x} – \sqrt {5 – 2x} }}{x}\)
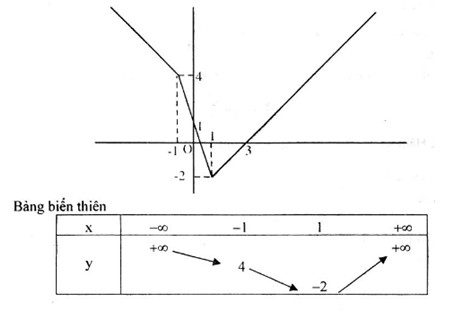
3.. Vẽ đồ thị và lập bẳng biến thiên của hàm số \(y = 2\left| {x – 1} \right| – \left| {x + 1} \right|\)

1. Hàm số \(y = \dfrac{{2x – 1}}{{x + 1}}\) có tập xác định \(D = \mathbb{R}\backslash \left\{ { – 1} \right\}\) .
Lấy \({x_1},{x_2} \in D,{x_1} \ne {x_2}\) .
Lập tỉ số
\(\begin{array}{l}k = \dfrac{{f\left( {{x_1}} \right) – f\left( {{x_2}} \right)}}{{{x_2} – {x_1}}}\\\;\; = \dfrac{{\dfrac{{2{x_2} – 1}}{{{x_2} + 1}} – \dfrac{{2{x_1} – 1}}{{{x_1} + 1}}}}{{{x_2} – {x_1}}}\\\;\; = \dfrac{{\left( {2{x_2} – 1} \right)\left( {{x_1} + 1} \right) – \left( {2{x_1} – 1} \right)\left( {{x_2} + 1} \right)}}{{\left( {{x_2} – {x_1}} \right)\left( {{x_1} + 1} \right)\left( {{x_2} + 1} \right)}}\end{array}\)
Advertisements (Quảng cáo)
\(\begin{array}{l}\;\; = \dfrac{{3{x_2} – 3{x_1}}}{{\left( {{x_2} – {x_1}} \right)\left( {{x_1} + 1} \right)\left( {{x_2} + 1} \right)}}\\ \;\;= \dfrac{3}{{\left( {{x_1} + 1} \right)\left( {{x_2} + 1} \right)}}\end{array}\)
Nếu \({x_1},{x_2} \in \left( { – \infty ; – 1} \right)\) thì \({x_1} < – 1,{x_2} < – 1\) .Suy ra \({x_1} + 1 < 0,{x_2} + 1 < 0\) . Do đó k > 0. Vậy hàm số đồng biến trên khoảng \(\left( { – \infty ; – 1} \right)\) .
Nếu \({x_1},{x_2} \in \left( { – 1; + \infty } \right)\) thì \({x_1} > – 1,{x_2} > – 1\). Suy ra \({x_1} + 1 > 0,{x_2} + 1 > 0\) . Do đó k > 0. Vây hàm số đồng biến trên khoảng \(\left( { – 1; + \infty } \right)\)
2. Hàm số \(f(x) = \dfrac{{\sqrt {5 + 2x} – \sqrt {5 – 2x} }}{x}\) được xác định khi và chỉ khi
\(\left\{ \begin{array}{l}5 + 2x \ge 0\\5 – 2x \ge 0\\x \ne 0\end{array} \right. \Leftrightarrow \left\{ \begin{array}{l}x \ge – \dfrac{5}{2}\\x \le \dfrac{5}{2}\\x \ne 0\end{array} \right.\\ \Leftrightarrow \left\{ \begin{array}{l} – \dfrac{5}{2} \le x \le \dfrac{5}{2}\\x \ne 0\end{array} \right.\)
Vậy hàm số có tập xác định \(D = \left[ { – \dfrac{5}{2};0} \right) \cup \left( {0;\dfrac{5}{2}} \right]\) .
Advertisements (Quảng cáo)
Với mọi \(x \in D\) ta có
\( – x \in D\)
\(\begin{array}{l}f\left( { – x} \right) = \dfrac{{\sqrt {5 – 2x} – \sqrt {5 + 2x} }}{{ – x}}\\{\rm{ }} = \dfrac{{\sqrt {5 + 2x} – \sqrt {5 – 2x} }}{x} = f(x)\end{array}\)
Vậy hàm số \(f(x) = \dfrac{{\sqrt {5 + 2x} – \sqrt {5 – 2x} }}{x}\) là hàm số chẵn.
3. Ta có:
\(y = \left\{ \begin{array}{l}2\left( { – x + 1} \right) – \left( { – x – 1} \right){\rm{\; khi\; x < – 1}}\\{\rm{2}}\left( { – x + 1} \right) – \left( {x + 1} \right){\rm{ khi – 1}} \le {\rm{x}} \le {\rm{1}}\\{\rm{2}}\left( {x – 1} \right) – \left( {x + 1} \right){\rm{ khi x > 1}}\end{array} \right.\\\;\; = \left\{ \begin{array}{l} – x + 3{\rm{ khi x < – 1}}\\{\rm{ – 3x + 1 khi – 1}} \le {\rm{x}} \le {\rm{1}}\\{\rm{x – 3 khi x > 1}}\end{array} \right.\)
Đồ thị