Bài 31: Gọi \(S\) là diện tích và \(R\) là bán kính đường tròn ngoại tiếp tam giác \(ABC\). Chứng minh rằng \(S = 2{R^2}\sin A\sin B\sin C\).

Áp dụng công thức tính diện tích và định lí sin trong tam giác \(ABC\) .Ta có
\(\eqalign{
& S = {{abc} \over {4R}} = {{(2R\sin A).(2R\sin B).(2R\sin C)} \over {4R}} \cr
& \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = 2{R^2}\sin A\sin B\sin C \cr} \)
Bài 32: Chứng minh rằng diện tích của một tứ giác bằng nửa tích hai đường chéo và sin của góc hợp bởi hai đường chéo đó.

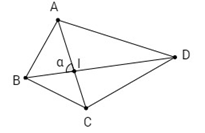
Gọi \(I\) là giao điểm của hai đường chéo \(AC, BD\) và \(\widehat {AIB} = \alpha \).
Ta có \({S_{ABI}} = {1 \over 2}AI.BI.\sin \alpha \,\,,\,\,\,{S_{ADI}} = {1 \over 2}AI.DI.\sin ({180^0} – \alpha ) = \,{1 \over 2}AI.DI.\sin \alpha \,\)
Suy ra \({S_{ABD}} = {S_{ABI}} + {S_{ADI}} = {1 \over 2}AI.(BI + DI).\sin \alpha = {1 \over 2}AI.BD.\sin \alpha \)
Tương tự ta suy ra \({S_{BCD}} = {S_{BIC}} + {S_{CDI}} = {1 \over 2}CI.BD.\sin \alpha \)
Từ đó suy ra
\({S_{ABCD}} = {S_{ABD}} + {S_{BCD}} = {1 \over 2}.BD.(AI + CI).\sin \alpha = {1 \over 2}.BD.AC.\sin \alpha. \)
Bài 33: Giải tam giác \(ABC\), biết
Advertisements (Quảng cáo)
a) \(c = 14,\,\widehat A = {60^0},\,\widehat B = {40^0}\);
b) \(b = 4,5,\,\widehat A = {30^0},\,\widehat C = {75^0}\);
c) \(c = 35,\,\widehat A = {40^0},\,\widehat C = {120^0}\);
d) \(a = 137,5;\;\widehat B = {83^0},\,\widehat C = {57^0}\).

a) Ta có \(\widehat C = {180^0} – {60^0} – {40^0} = {80^0}\)
Áp dụng định lí sin :
\(\eqalign{
& \,\,\,\,\,\,{a \over {\sin A}} = {b \over {\sin B}} = {c \over {\sin C}} = {{14} \over {{\mathop{\rm s}\nolimits} {\rm{in8}}{{\rm{0}}^0}}}\,\,\,\, \Rightarrow \,\,a = {{14} \over {{\mathop{\rm s}\nolimits} {\rm{in8}}{{\rm{0}}^0}}}.\sin {60^0} \approx 12,3 \cr
& \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,b = {{14} \over {{\mathop{\rm s}\nolimits} {\rm{in8}}{{\rm{0}}^0}}}.\sin {40^0} \approx 9,1 \cr} \)
b) Ta có \(\widehat B = {180^0} – {30^0} – {75^0} = {75^0}\)
Advertisements (Quảng cáo)
Áp dụng định lí sin
\(\eqalign{
& \,\,\,\,\,\,{a \over {\sin A}} = {b \over {\sin B}} = {c \over {\sin C}} = {{4,5} \over {{\mathop{\rm s}\nolimits} {\rm{in7}}{{\rm{5}}^0}}}\,\,\, \Rightarrow \,\,a = {{4,5} \over {{\mathop{\rm s}\nolimits} {\rm{in7}}{{\rm{5}}^0}}}.\sin {30^0} \approx 2,3 \cr
& \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,c = {{4,5} \over {{\mathop{\rm s}\nolimits} {\rm{in7}}{{\rm{5}}^0}}}.\sin {75^0} = 4,5 \cr} \)
c) Ta có \(\widehat B = {180^0} – {120^0} – {40^0} = {20^0}\)
Áp dụng định lí sin :
\(\eqalign{
& \,\,\,\,\,\,{a \over {\sin A}} = {b \over {\sin B}} = {c \over {\sin C}} = {{35} \over {{\mathop{\rm s}\nolimits} {\rm{in12}}{{\rm{0}}^0}}}\,\,\,\,\, \Rightarrow \,\,a = {{35} \over {{\mathop{\rm s}\nolimits} {\rm{in12}}{{\rm{0}}^0}}}.\sin {40^0} \approx 26 \cr
& \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,b = {{35} \over {{\mathop{\rm s}\nolimits} {\rm{in12}}{{\rm{0}}^0}}}.\sin {20^0} \approx 13,8 \cr} \)
d) Ta có \(\widehat A = {180^0} – {83^0} – {57^0} = {40^0}\)
Áp dụng định lí sin :
\(\eqalign{
& \,\,\,\,\,\,{a \over {\sin A}} = {b \over {\sin B}} = {c \over {\sin C}} = {{137,5} \over {{\mathop{\rm s}\nolimits} {\rm{in4}}{{\rm{0}}^0}}}\,\,\,\, \Rightarrow \,\,b = {{137,5} \over {{\mathop{\rm s}\nolimits} {\rm{in4}}{{\rm{0}}^0}}}.\sin {83^0} \approx 212,3 \cr
& \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,c = {{137,5} \over {{\mathop{\rm s}\nolimits} {\rm{in4}}{{\rm{0}}^0}}}.\sin {57^0} \approx 179,4 \cr} \)
Bài 34: Giải tam giác \(ABC\), biết
a) \(a = 6,3,\,\,b = 6,3,\,\,\widehat C = {54^0}\);
b) \(b = 32,\,c = 45,\,\widehat A = {87^0}\);
c) \(a = 7,\,\,b = 23,\,\,\widehat C = {130^0}\).
a) \(ABC\) là tam giác cân tại \(C\) \( \Rightarrow \,\,\widehat A = \widehat B = {{{{180}^0} – {{54}^0}} \over 2} = {63^0}\). Áp dụng định lí sin ta có
\(\,\,\,\,\,\,{a \over {\sin A}} = {c \over {\sin C}} = {{6,3} \over {{\mathop{\rm s}\nolimits} {\rm{in6}}{{\rm{3}}^0}}}\,\, \Rightarrow \,\,c = {{6,3} \over {{\mathop{\rm s}\nolimits} {\rm{in6}}{{\rm{3}}^0}}}.\sin {54^0} \approx 5,7\)
b) Áp dụng định lí cosin ta có
\(\eqalign{
& {a^2} = {b^2} + {c^2} – 2bc.\cos A \cr
& \,\,\,\,\,\, = {32^2} + {45^2} – 2.32.45.\cos {87^0} \approx 2898,27 \cr
& \Rightarrow a \approx 53,8 \cr} \)
Áp dụng định lí sin ta có
\(\eqalign{
& \,\,\,\,\,\,{a \over {\sin A}} = {b \over {\sin B}}\,\, \Rightarrow \,\,\sin B = {{b\sin A} \over a} = {{32.\sin {{87}^0}} \over {53,8}} \approx 0,6 \cr
& \Rightarrow \,\,\widehat B \approx {36^0}\,,\,\,\widehat C \approx {57^0} \cr} \)
c) Áp dụng định lí cosin ta có
\(\eqalign{
& {c^2} = {a^2} + {b^2} – 2ab.\cos C \cr
& \,\,\,\,\,\, = {7^2} + {23^2} – 2.7.23.\cos {130^0} \approx 785 \cr
& \Rightarrow c \approx 28 \cr
& \cos A = {{{b^2} + {c^2} – {a^2}} \over {2bc}} = {{{{23}^2} + {{28}^2} – {7^2}} \over {2.23.28}} \approx 0,98 \cr
& \Rightarrow \,\,\widehat A = {11^0}\,,\,\,\widehat B = {39^0} \cr} \)

